Lupine Publishers | Civil Engineering Research Journal
This paper reports on a study carried out to optimize the locations
of multiple discharge points in a receiving stream, Amadi
creek, so as to minimize the impact of oxygen demanding resources (BOD)
on water quality. The study evaluated the water
quality changes as a result of the increasing human and industrial
activities around the creek. Water quality standards require
the maintenance of dissolved oxygen (DO) concentration of 5mg/l or more
at any time in streams. However practical analysis of
the water samples from Amadi creek reveal a DO level as low as 2.3mg/l.
The DO deficit was computed from data generated by
sampling DO concentrations along the creek from various points of waste
discharge downstream while the BOD of the stream was
determined by monitoring BOD of samples obtained along the creek. The
study also identified and quantified the amount of effluent
entering the creek from various point sources. The DO deficit equations
are solved by the methods of simple calculus (classical
optimization), which simplifies the mathematical solution of the model
equations by avoiding difficult to evaluate integrals Two
scenarios were identified and used to investigate the effect of BOD on
the DO level in the stream, using mathematical simulation
techniques. Simulation results show that to ensure minimum impact of BOD
on water quality waste discharge locations should be
placed at the optimal locations of 10015.382m and 6992.282m upstream and
downstream waste discharge points respectively, at
an optimum DO deficit of 4.135mg/l for case 1.
For case2, the waste discharge locations are to be placed at optimal
locations 40995.43m, 30665.17m, 41233.69m upstream
and downstream waste discharge points respectively at an optimum DO
deficit of 4.567mg/l. This means that if a new waste input is
proposed for a stream its BOD input and its proposed location with
respect to other inputs are important in order to determine the
effect on the DO level in the stream Discharges from the second
treatment plant would result in decreased dissolved oxygen level for
a substantial distance downstream. This can have significant effects for
streams and rivers with many influent waste streams over
their course, as the dissolved oxygen (DO) will not have a chance to
recover between each influent stream, resulting in significantly
depressed oxygen levels .The dissolved oxygen (DO) deficit becomes zero
at approximately the same distance downstream for both
cases, though the two point source discharge case (case2) shows a higher
short term DO deficit. This can cause problems if they DO
concentration drops below the stipulated levels for the creek, leading
to possible death of fish and other aquatic lives. It is therefore
recommended that industrial establishments planning to site their
treatment facilities along rivers or streams should be compelled
to discharge their waste stream in compliance with the optimal locations
with respect to any existing plant, so as to avoid undue
dissolved oxygen (DO) depletion.
Keywords: Point Sources; Effluent; Discharge Point; Impact, Creek; Optimal Location; Sampling
Introduction
Modeling the impact of Biological Oxygen Demand (BOD) on
water quality is an important part of the permitting process for new
resources Masters [1]; Agunwamba et al. [2]; Peavy et al. [3]. Many
rivers and streams in Port-Harcourt metropolis, Nigeria as a whole
and indeed all over the world have suffered from dissolved oxygen
(DO) deficit, which is very crucial to survival of aquatic life. Stream
models can help determine the maximum amount of additional
BOD that will be allowed, which, in turn, affects facility siting
decisions and the extent of on-site waste water treatment that will
be required Agunwamba et al. [2]; Mcbride [4] ; Ezeilo et al. [5];
Dobbins [6] .The amount of dissolved oxygen (DO) in water is one
of the most commonly used indicators of a rivers health ( Ezeilo
et.al, 2012 ). As DO drops below 4 or 5 mg/l, the forms of life that
can survive begin to be reduced. In the extreme case when hypoxic
conditions (0<DO<5mg/l) exist, most higher forms of life are
killed
or driven off. Among the factors affecting the DO available in a
stream are BOD, which account for the oxygen demanding wastes
Brown [7]; Ezeilo et al. [8], Ezeilo et al. [9]. Photosynthesis also
affect DO. Algae and other aquatic plants add DO during the daytime
hours, while photosynthesis is occurring, but at night their
continued respiration draws it down again. The net effect is a diurnal
variation that can lead to elevated levels of DO in the late afternoon
and depressed concentrations at night. For a lake or a slow-moving
stream that is already overloaded with BOD and choked with
algae, it is not unusual for respiration to cause offensive, anaerobic
conditions late at night, even though the river seems fine during the
day. Other factors which would affect DO availability in a stream
include, accumulated sludge along the bottom, tributaries, which
mix with those of the mainstream. etc. Water quality modeling in
a river has developed from the pioneering effort of Streeter and
Phelps [10], who proposed a mathematical model demonstrating
how DO in the Ohio River decreased with downstream distance due
to degradation of soluble organic BOD.
According to Yudianto et al. [11] the simplest manifestation
of this equation is usually applied for a river reach characterized
by plug flow system with constant hydrology and geometry under
steady state condition, as occurred in Amadi creek. For a large
river or estuary, considerable longitudinal dispersion influences
the phenomenon of DO and BOD distribution and so the governing
equations becomes a partial differential equation. However, the
effect of dispersion on DO and BOD in small rivers, like Amadi
Creek, used in this study, is negligible Li [12]. Water collected for
sampling is discharged into Amadi creek without any treatment
as point source. Therefore, specifically Amadi creek is modeled
with single point source of BOD in this study. Much research has
been done on the area of DO depletion in water bodies, providing
information on critical deficit, critical distance, and minimum DO
concentration, but none of these studies has attempted to optimize
the waste discharge locations for minimum impact of oxygen
demanding resources (BOD) on water quality. This would have
enabled us to establish an optimum deficit and optimum discharge
locations for minimum impact of oxygen demanding resources on
water quality. Such a study has been undertaken in Amadi Creek.
This research was therefore carried out to identify and quantify
the amount of waste water effluent entering the creek and evaluate
the impact of these oxygen demanding wastes on water quality.
A novel approach that will help minimize stream pollution when
there are many industries discharging waste water into a stream
is presented. Also, the determination of points of maximum DO
deficit in case of multiple discharges along a stream using classical
optimization technique is discussed.
Brief Description of the Study Area
The Amadi Creek has the Bonny river as its major source. It
flows from Okrika down to Mini-Ewa, Rumuobiakani through Woji,
Oginigba, Okujagu communities and back to the Bonny river where
it empties out into the Atlantic oc ean. Amadi creek is located in
Obio-Akpor Local Government Area and is host to several industries
and factories as well as the popular Port-Harcourt city abbatoir
(Slaughter). A sketch of Amadi Creek showing point sources of
pollution are shown in Figure 1.
Figure 1: A sketch of Amadi Creek showing point-sources of pollution (Agunwamba et al, 2006).

Methodology
Data was generated by sampling DO concentrations along the
creek aboard a boat from various waste discharge locations and
monitoring the BOD of samples obtained along the creek. The
water temperature and pH was also determined. Other parameters
determined include creek depth, width, flow velocity and flow rates.
The BOD and DO were determined following the procedures given
in the standard methods ( Apha,1998). Samples were collected
with winkler bottles at intervals downstream, sealed to exclude
air bubbles and sent to the laboratory for analysis. The depth were
measured by dropping a loaded tape to the bottom of the creek,
while the width was measured by stretching a tape across the creek.
Temperature was measured on site using a clinical thermometer,
while velocity was determined with a current meter.
Theoretical Formulation
Case 1 – One Source of Waste Water Discharge (Figure 2)
Figure 2: Two reach model of a stream with a single point-source.

The dissolved oxygen deficit along the reaches are:
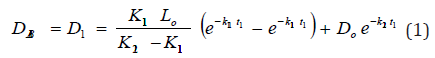
where, Lo is ultimate BOD concentration upstream effluent
discharge, Do initial Do concentration upstream effluent discharge
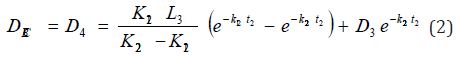
The concentrations just downstream are computed by a mass
balance as;
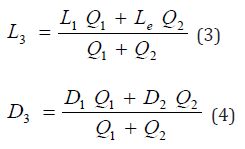
where, Q 1, Q 2 are the stream and effluent discharges
respectively. L 1, L 3 are BOD of stream upstream and downstream
respectively, L 2 is BOD of Effluent discharge, D 1, 3, are the DO
concentrations upstream and downstream respectively, D2, is the DO
concentration of Effluent discharge. In the Streeter and Phelps
derivation the differential for L is assumed as
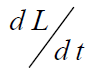
which integrates
to;
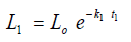
Substituting equations (3) and (4) into eq (2), gives
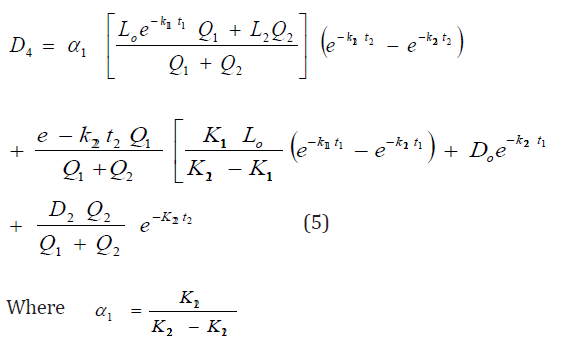
Case 2 – Two Sources of Waste Water Discharge (Figure 3)
Figure 3: Two-reach model of a stream with two-point sources.
The dissolved oxygen deficit along the reach CD, gives;

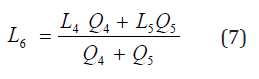

where, Q4, Q5, are the stream and Effluent discharge respectively,
L4, L6, are BOD upstream and downstream respectively, L 5 is BOD
of Effluent discharge, D4, D5 are the DO concentrations of stream
and Effluent respectively.
Substituting equations (7) and equation (8) into equation (6)
gives:
 Assumptions
Assumptions
a) The main assumption in the formulation of the equations
is that the river reach is characterized by plug flow system with
constant hydrology and geometry under steady state condition and
is traveling at a constant speed (u).
b) It is also assumed that water temperature is constant
throughout the stream. Mixing of different temperature streams is
not accounted for.
c) It is assumed that there is a constant discharge of waste
water into the creek and also that the waste water is discharged
into Amadi creek without any treatment as point source. Therefore,
specifically Amadi creek is modeled with single point source of BOD
in this study.
Optimization Problem
The problem of searching for the optimal waste discharge
locations, for minimum impact on water quality may be expressed
(Figures 2 & 3) as;

Subject to, t
1>0, t
2>0
where, X
1 and ho are the optimal waste discharge locations
Equations (5) and (6) represents the mathematical models
for the stated problem. This is an optimization (maximization)
problem Nwaigwe [13]. The desired solution of the above problem
involves the search for the optimal values of the waste discharge
locations i.e. the optimal determination of the values of x1 and ho
for each waste discharge point. This can be solved by the method of
simple calculus as follows;
Case 1: The derivative of equation (5) when
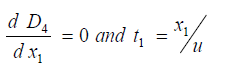
is
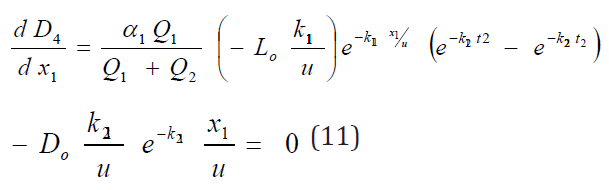
Where
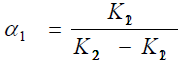
The derivative of e. q (5) when
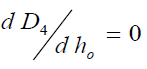
is
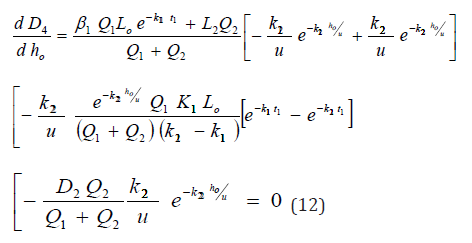
Where

Hence the optimal locations X1 and ho at which the waste
discharge locations will be placed for minimum impact on water
quality are obtained by solving equations (11) and (12) as;


where X2 is the downstream location, and u is the average
stream velocity, while t3 is the time of travel.
The derivative of e q (10) when
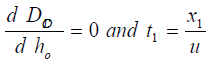


The derivative of eq when
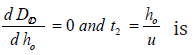
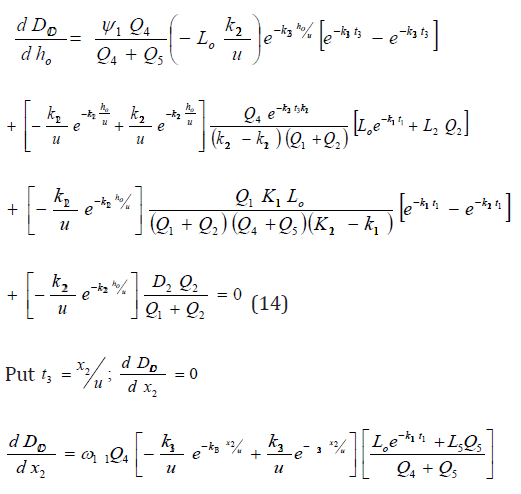
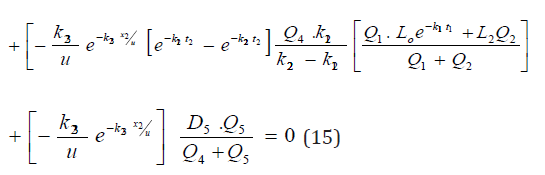
Hence the optimal locations X1, ho and X2 at which the waste
discharge locations will be placed for minimum impact on water
quality are obtained by solving equations (13), (14), (15) as;
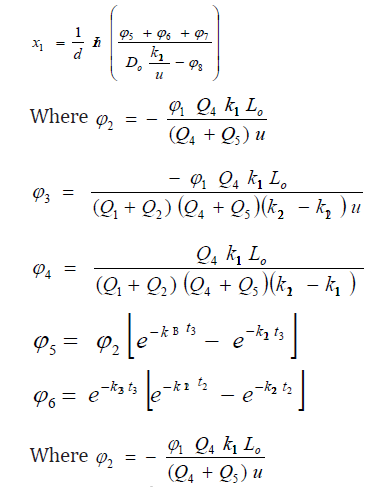



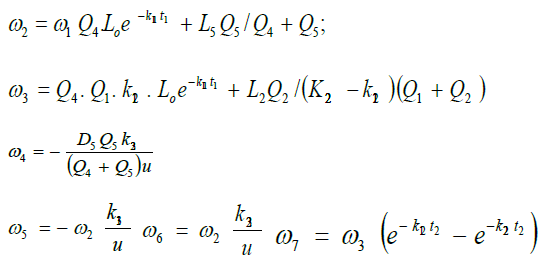
x
1, ho and x
2 are the optimal waste discharge locations and Q
4
, Q
5, are the Stream and Effluent discharges respectively. L
5 and
D
5 are the Effluent BOD and DO concentrations respectively. K
13,
k
23 are the de oxygenation and re aeration rates respectively. As
previously mentioned when eqn (1) and eqn (6) are assumed to be
steady state, time (or distance) is the only independent variable.
Equations (5) and (6) are the mathematical models for the problem
of optimization of the waste discharge locations in rivers. These
equations can be solved for the root t by a numerical root finding
method in a software package such as MATHCAD or MATEMATICA.
The value of t is then substituted into eqn (5) or eqn (6) to calculate
the optimum DO deficit. An alternative procedure to finding the
optimum DO deficit is to apply a series of times or velocities in eqn
(5) and eqn (6) and record the value of the optimum DO deficit
and the times or velocities to which it corresponds Nwaigwe [13].
Since the DO equations contain both DO deficit and t (which is a
function of DO), the solution is thus arrived at by iteration using
the Newton- Raphson method with the help of a developed VISUAL
BASIC Programme.
Application of Models
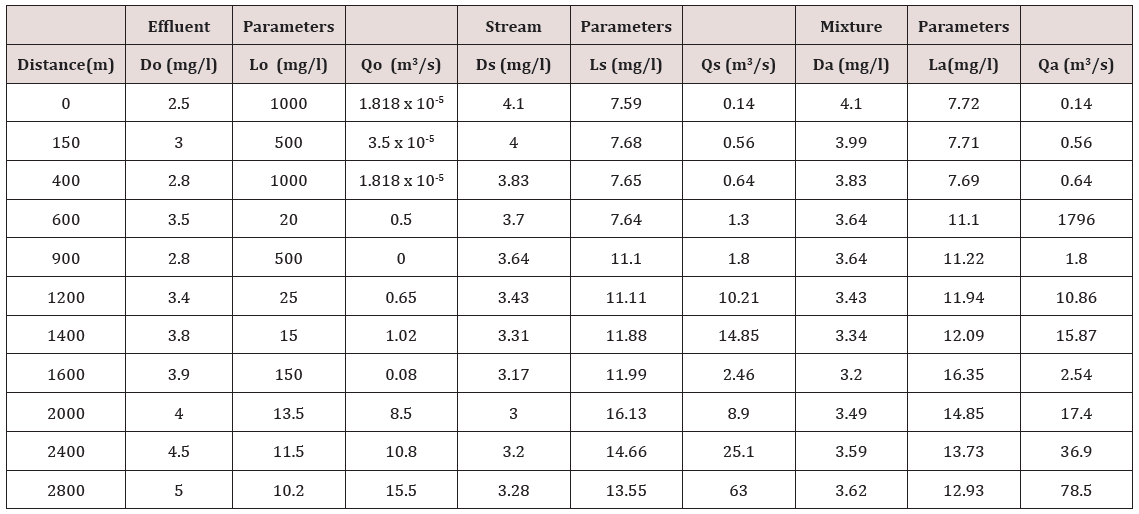
The developed models were applied to the water quality data
for Amadi Creek (Table 1). The input data for simulations of the two
case studies are;
Table 1: Amadi Creekwater Quality Parameters Agunwamba et al. [2].
Case 1: Q1 = 0.139m
3/s, Q2 = 0.5m
3/s, Lo = 7.59 mg/l, Do = 4.1
mg/l
k11 = 0.1/day, k12 = 0.17/day, L2 = 20mg/l, k21 =0.17/day k22
= 0.23/day.
Case 2: Q5 = 0.65m
3/s, L2 = 25 mg/l, D5 = 3.4 mg/l, k13 = 0.26/
day, k23 = 0.42/day
Substituting the above values in the above equations gives
the optimal locations X1 and ho as10015.382m and 6992.282m
respectively, at an optimum deficit of 4.135mg/l for case 1.Thus
the model predicts that at an optimum deficit of 4.135mg/l the
waste discharge locations would have to be placed at 10015.382m
and 6992.282m upstream and downstream waste discharge
locations respectively for minimum impact on water quality. For
case 2, substituting these values in the above equations results
in an optimum DO deficit of 4.567mg/l at optimal locations of
41233.43m, 40995.17m, 30665.69m upstream and downstream
waste discharge locations respectively, to ensure minimum impact
on water quality. The DO, BOD and temperature of the mixture
effluent with creek water was obtained.
From
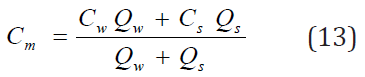
where Cm represents concentration of any parameter such as
DO, BOD and water temperature at the point effluent mixes with
creek water. The subscript denotes stream (or creek) and waste
water respectively and Qs is the stream flow rate and Qw is the
waste water flow rate. In order to convert BOD5 to ultimate BOD
(Lo), using the first order decay rate, an extrapolation can be made
according to Agunwamba et al. [2], as follows:

Effect of Flow conditions on Single Point Source Discharge
Figure 4: Effect of flow conditions on a single point waste discharge.
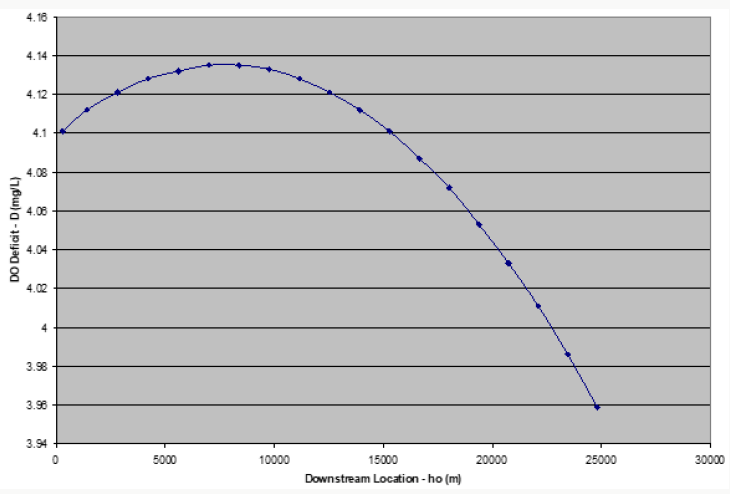
The result of the single point source case is presented in
Figure 4. As expected the DO deficit curve rises to the point of
optimum (maximum) deficit as the BOD is being degraded, and
then start decreasing as the effect of the waste stream is felt
approximately10000 meters downstream of the outfall. Here the
stream becomes super-saturated due to the increased production
of oxygen. This increased DO concentration may have been due to
the activities of phytoplankton species living in the water (Ezeilo
et.al 2012), and enhanced re aeration due to the optimum deficit
of 4.135 mg/l. At this point, which corresponds to the point of
maximum deficit, the stream undergoes a high level of re aeration.
This is because DO deficit is the driving force for the replenishment
of oxygen in polluted waters Sakalauskiene [14]. Thus, the greater
the deficit, the greater the transfer of oxygen into the water
Agunwamba [2]. After the point of maximum deficit, and high re
aeration, the stream once again experiences a fall in the deficit
as we move downstream, due to lesser concentration of the BOD,
leading to improved DO concentrations, and so the DO equilibrates
at a much higher concentration than in the two-point case.
However, if there are other point sources downstream from the
sewage treatment plant, the combined effect could cause significant
depression of the stream DO concentration.
Effect of Flow Conditions on Two Sequential Point
Source Discharge
The result of the two-point source discharge is presented
graphically in Figure 5. They show the combined effect of a second
sewage treatment plant some distance downstream. This second
outfall is located in the region affected by the first out fall. The
result shows a lowered DO deficit curve, shifting appreciably by
the remaining DO deficit from the first outfall. This may have been
due to increase in stream flow from the addition of the first out
flow Ezeilo et al. [3]. This is also seen in the small change in the
slope curve at the point of discharge. The second effluent stream
results in a lowered DO concentration further downstream than
for the single point case .This drop in the DO concentration could
cause problems adjacent to the outfall if they DO concentration
fall below the minimum standards set for the stream as observed
by Brown [5] Due to increased effluent waste stream, very little
oxygen is retained in the stream, resulting in a depressed DO deficit
curve .Additionally, due to increased velocity, the travel time has
significantly decreased and the resultant effect is a depressed DO
concentration farther downstream than for the single point source
case.
Figure 5: Effect of flow conditions on a single point waste discharge.

Conclusions and Recommendations
The dissolved oxygen (DO) deficit is dependent on the distance
between multiple waste stream inputs (waste discharge points).
This means that if a new waste input ( a new sewage treatment
plant for example) is proposed for a stream or creek, both its BOD
input and the proposed location with respect to other inputs are
important in order to determine the effects on the dissolved oxygen
(DO) level in the stream Discharges from the second treatment
plant would result in decreased dissolved oxygen level for a
substantial distance downstream. This can have significant effects
for streams and rivers with many influent waste streams over
their course, as the dissolved oxygen (DO) will not have a chance
to recover between each influent stream, resulting in significantly
depressed oxygen levels .The dissolved oxygen (DO) deficit
becomes zero at approximately the same distance downstream
for both cases, though the two point source discharge case (case2)
shows a higher short term DO deficit. This can cause problems if
they DO concentration drops below the stipulated levels for the
creek, leading to possible death of fish and other aquatic lives. It is
recommended that industrial establishments planning to site their
treatment facilities along rivers or streams should be compelled
to discharge their waste stream in compliance with the optimal
locations with respect to any existing plant, so as to avoid undue
dissolved oxygen (DO) depletion.
For more
Lupine Publishers Open Access Journals Please visit our website:
http://www.lupinepublishers.com/
For more
Civil Engineering Research Journal articles Please Click Here:
https://lupinepublishers.com/civil-engineering-journal/
To Know More About
Open Access Publishers Please Click on Lupine Publishers
https://lupinepublishers.us/
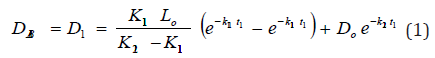
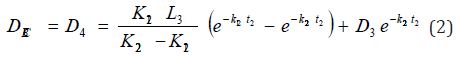
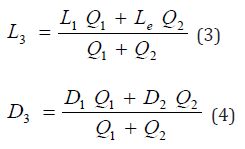
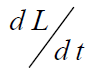 which integrates
to;
which integrates
to;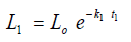
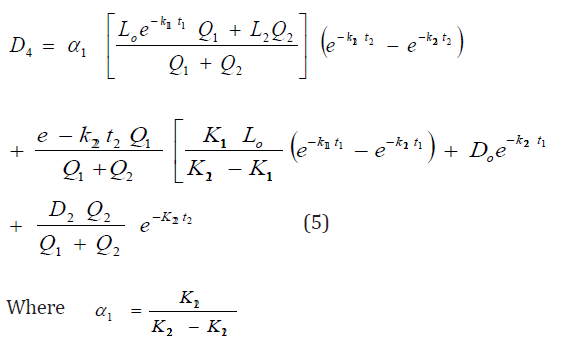

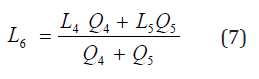



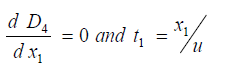 is
is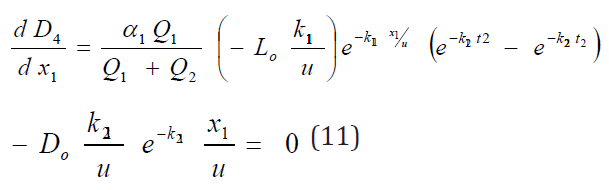
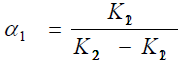
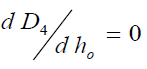 is
is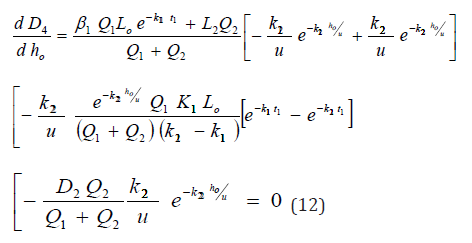



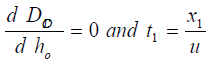


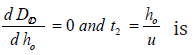
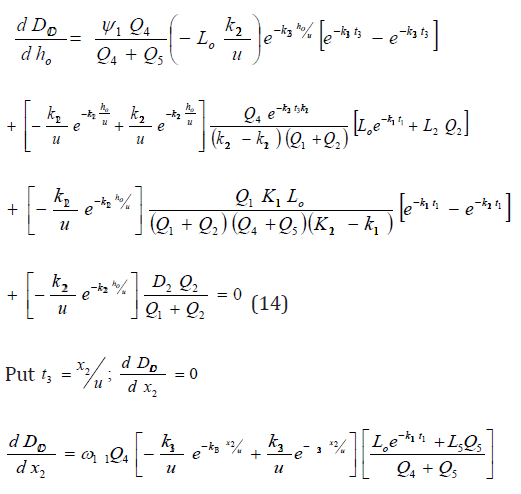
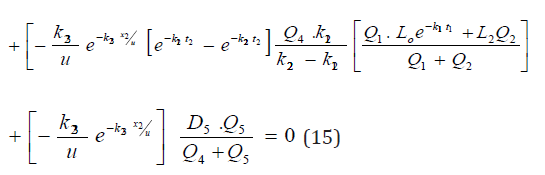
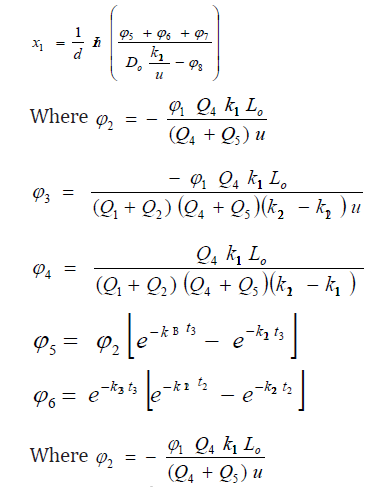



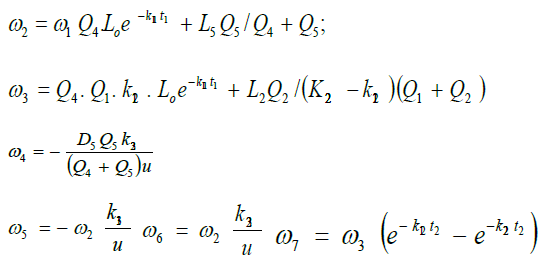
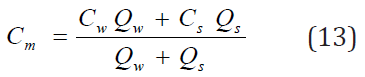











No comments:
Post a Comment